Published On:Tuesday, 20 December 2011
Posted by Muhammad Atif Saeed
Solving Literal Equations
Sometimes you have a formula, such as something from geometry, and you need to solve for some variable other than the "standard" one. For instance, the formula for the perimeter P of a square with sides of length s is P = 4s. You might need to solve this equation for s, so you can plug in a perimeter and figure out the side length.
This process of solving a formula for a given variable is called "solving literal equations". One of the dictionary definitions of "literal" is "related to or being comprised of letters", and variables are sometimes referred to as literals. So "solving literal equations" seems to be another way of saying "taking an equation with lots of letters, and solving for one letter in particular."
At first glance, these exercises appear to be much worse than your usual solving exercises, but they really aren't that bad. You pretty much do what you've done all along for solving linear equations and other sorts of equation; the only substantial difference is that, due to all the variables, you won't be able to simplify your answers as much as you're used to. Here's how "solving literal equations" works:
- Solve A = bh for b If they'd asked me to solve 3 = 2b for b, I'd have divided both sides by 2. Following the same reasoning, I get:
- Solve d = rt for r Copyright © Elizabeth Stapel 2002-2011 All Rights Reserved
- Solve P = 2l + 2w for w If they'd asked me to solve 3 = 2 + 2w for w, I'd have moved the "free" 2 to the other side, and then divided through by the 2 on the variable. Following the same reasoning, I get:
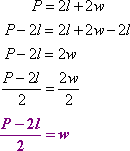
- Solve Q = (c + d)/2 for d
![2Q = (2/1)[(c + d)/2]](http://www.purplemath.com/modules/solvlin/slvlit04.gif) 2Q = c + d
2Q – c = c + d – c
2Q – c = d
2Q = c + d
2Q – c = c + d – c
2Q – c = d - Solve V = 3k/t for t If they'd asked me to solve 5 = 3 / t for t, I'd have multiplied through by t, and then divided both sides by 5. Following the same reasoning, I get:
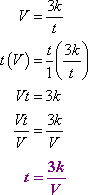
This next exercise requires a little "trick" to solve it:
- Solve Q = 3a + 5ac for a
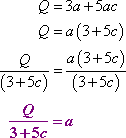
The "trick" came in the second line, where I factored out the a. This technique doesn't come up often, but it's just about guaranteed to come up in your homework once or twice, and almost-certainly on your next test, precisely because so many students don't see the "trick". So keep in mind: When you can't isolate the desired variable because it is a factor in two or more terms, collect those terms together on one side of the "equals" sign, factor out the desired variable, and then divide off whatever is left.
- Solve A = ( 1/2 )ah – ( 1/2 )bh for h
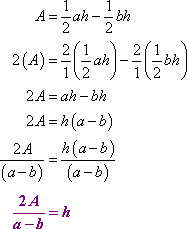
This example used the same "trick" as the previous one. In the fourth line, I factored out the h. You should expect to need to know how to do this!
- The area A of a sector (a pie-wedge-shaped section) of a circle is given by:
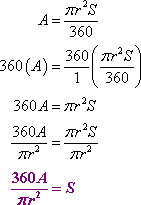
- What is the slope of the line with equation 3x + 2y = 8?
- In order to find the slope, it is simplest to put this line equation into slope-intercept form. If I rearrange this line to be in the form "y = mx + b", it will be easy to read off the slope m. So I'll solve: Copyright © Elizabeth Stapel 2002-2011 All Rights Reserved
- 3x + 2y = 8
2y = –3x + 8
y = ( –3/2 ) x + 4
- Find the slope and y-intercept of the line with equation 2x – y = 5.
- I'll solve for "y =":
- 2x – y = 5
2x = y + 5
2x – 5 = y
- the slope is m = 2 and the y-intercept is b = –5.
- Find the slope and y-intercept of the line with equation x – 2y = 5.
- I'll solve for "y =":
- x – 2y = 5
x = 2y + 5
x – 5 = 2y
( 1/2 ) x – ( 5/2 ) = y
- the slope is m = 1/2 and the y-intercept is b = –5/2 .
- Find the slope and y-intercept of the line with equation 4x + 5y = 12.
- I'll solve for "y =":
- 4x + 5y = 12
5y = – 4x + 12
y = ( –4/5 ) x + ( 12/5 )
Don't let literal equations "throw" you. Solving literal equations is just like solving linear (and other sorts of) equations, except that the answers don't simplify as much. The techniques involved are otherwise exactly the same. Just take your time and be sure to write out all your steps clearly









