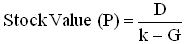Published On:Thursday, 8 December 2011
Posted by Muhammad Atif Saeed
Roots and Radicals
Introduction to Roots and Radicals
The symbol
is called a radical sign and is used to designate square root. To designate cube root, a small three is placed above the radical sign, 
. When two radical signs are next to each other, they automatically mean that the two are multiplied. The multiplication sign may be omitted. Note that the square root of a negative number is not possible within the real number system; a completely different system of imaginary numbers is used. The (so-called) imaginary numbers are multiples of the imaginary unit i. 
Simplifying Square Roots
Example 1
Simplify.- a, b
-
- If each variable is nonnegative (not a negative number),
- eIf each variable is nonnegative,
- fIf each variable is nonnegative,
- gIf each variable is nonnegative,
- hIf each variable is nonnegative,
- iIf each variable is nonnegative,
- jIf each variable is nonnegative,
Operations with Square Roots
You can perform a number of different operations with square roots. Some of these operations involve a single radical sign, while others can involve many radical signs. The rules governing these operations should be carefully reviewed.Under a single radical sign
You may perform operations under a single radical sign.Example 1
Perform the operation indicated.
When radical values are alike
You can add or subtract square roots themselves only if the values under the radical sign are equal. Then simply add or subtract the coefficients (numbers in front of the radical sign) and keep the original number in the radical sign.Example 2
Perform the operation indicated.
. When radical values are different
You may not add or subtract different square roots.Example 3
Addition and subtraction of square roots after simplifying
Sometimes, after simplifying the square root(s), addition or subtraction becomes possible. Always simplify if possible.Example 4
Simplify and add.-
These cannot be added untilis simplified.
Now, because both are alike under the radical sign,
-
Try to simplify each one.
Now, because both are alike under the radical sign,
Products of nonnegative roots
Remember that in multiplication of roots, the multiplication sign may be omitted. Always simplify the answer when possible.Example 5
Multiply.-
- If each variable is nonnegative,
- If each variable is nonnegative,
- If each variable is nonnegative,
-
Quotients of nonnegative roots
For all positive numbers,
Example 6
Divide. Leave all fractions with rational denominators.
Example 7
Divide. Leave all fractions with rational denominators.-
- First simplify
:
or
-
-
Example 8
